odd function integration|integral calculator : Tuguegarao For an odd function, I know that f(x) = - f(x). I'm trying to show that $\int^{a}_{-a} f(x) dx$ = 0. I've seen the proof where it splits the integral up into: . SULASOK.TV - The No.1 Best Pinay Sex & Pinay Porn Sites in terms of newest Trending and Viral. Watch free latest and Updated HD videos every day. Watch HD Pinay Porn, Pinay Scandals, Asian Porn, and Western Porn for free. Access a huge database of XXX Adult contents exclusively at SULASOK pornsite.
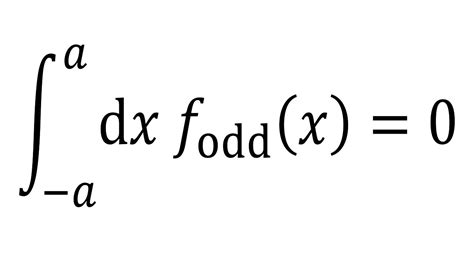
odd function integration,An odd function is one in which f (−x)= −f (x) f ( − x) = − f ( x) for all x x in the domain, and the graph of the function is symmetric about the origin. Integrals of even functions, when the limits of integration are from − a a to a a, involve two equal areas, because they are . Sometimes we can simplify a definite integral if we recognize that the function we’re integrating is an even function or an odd function. If the function is neither . For an odd function, I know that f(x) = - f(x). I'm trying to show that $\int^{a}_{-a} f(x) dx$ = 0. I've seen the proof where it splits the integral up into: .
Now, let’s dig into integrals of even and odd functions! Let f be an integrable function on some closed interval that is symmetric about zero — for example [ − a, a], for a ≥ 0. If f is .
Let $f$ be an odd function with a primitive on the open interval $\openint {-a} a$, where $a > 0$. Then the improper integral of $f$ on $\openint {-a} a$ is: $\ds .
An odd function is one in which \(f(−x)=−f(x)\) for all \(x\) in the domain, and the graph of the function is symmetric about the origin. Integrals of even functions, when the limits of . Example 1.2.11 Odd functions. Three examples of odd functions are \(f(x)=\sin x\text{,}\) \(f(x)=\tan x\) and \(f(x)=x^3\text{.}\) In fact, if \(f(x)\) is any odd power .Symmetry appears throughout mathematics in many different forms, and its use often leads to insights and efficiencies. Here we use the symmetry of a function to simplify integral calculations. Section 1.1 introduced .
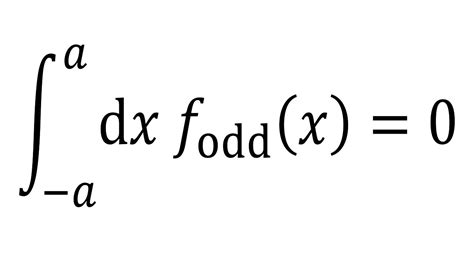
Integrating even and odd functions involves finding the definite integral of a function over a symmetric interval. For even functions, the integral over a symmetric interval is .integral calculatorIntegrating even and odd functions involves finding the definite integral of a function over a symmetric interval. For even functions, the integral over a symmetric interval is .Integrating Odd Functions on Symmetric Intervals. Since on one side of the origin the function is negative but has the same y-values as on the other side of the origin, areas under the curve cancel out over .2. Start by splitting the integral into two pieces, the part over negatives values of x and the part over positive values. ∫2 − 2f(x)dx = ∫0 − 2f(x)dx + ∫2 0f(x)dx. From here you can apply the definition of an even or odd function. Cite. answered Feb 13, .
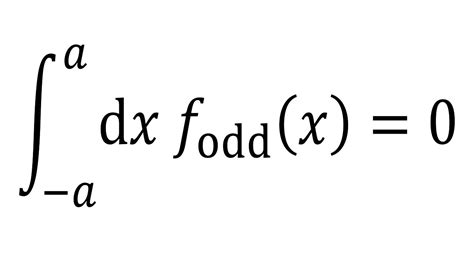
They are special types of functions. Even Functions. A function is "even" when: f(x) = f(−x) for all x In other words there is symmetry about the y-axis (like a reflection):. This is the curve f(x) = x 2 +1. They are . Theorem 1.2.1 Arithmetic of Integration. Let a, b and A, B, C be real numbers. Let the functions f(x) and g(x) be integrable on an interval that contains a and b. Then. (a) ∫b a(f(x) + g(x))dx = ∫b af(x)dx + ∫b ag(x)dx (b) ∫b a(f(x) − g(x))dx = ∫b af(x)dx − ∫b ag(x)dx (c) ∫b aCf(x)dx = C ⋅ ∫b af(x)dx. Combining these three . 1. This is true provided the integral is interpreted as Cauchy principal value. Since f f is odd, ∫A −A f(x)dx = 0 ∫ − A A f ( x) d x = 0. Hence, p. v.∫∞ −∞ f(x)dx = limA→∞∫A −A f(x)dx = 0 p. v. ∫ − ∞ ∞ f ( x) d x = lim A → ∞ ∫ − A A f ( x) d x = 0. There's also the implicit assumption that f f is .odd function integration integral calculator The integration of an odd function over a symmetric interval can be understood through some fundamental concepts in calculus and symmetry. An odd function is defined as a function f (x) f ( x) that satisfies the condition f (−x) = −f (x) f ( − x) = − f ( x) for all x x in the domain of the function. Graphically, odd functions exhibit .The theorem of “Integration of Even and Odd Functions” is a way to find integrals for odd and even functions. It’s a method that makes some challenging definite integrals easier to find. In order to use it, you have to be evaluating a function over an interval that’s either symmetric about the origin or symmetric about the y-axis [1]. Prove that a symmetric integral of an odd function is zero. This is true if the limits go from -a to a.I've got tons of integrals in my integrals playlist! C.
odd function integration|integral calculator
PH0 · rules of integration in calculus
PH1 · integral calculator
PH2 · Iba pa